difference between Simple and compound interest- formula and problems
After reading this post you will have clear understanding of what is simple interest and what is compound interest. Difference between simple interest and compound interest is explained with the help of solved examples.
Say, if the time period is one year
What is the simple interest
Interest paid is computed on the principal (the original sum of money). The simple interest remains the same in every time period provided that the rate of interest doesn't change.Say, if the time period is one year
Interest of 1st year = Interest of 2nd year = Interest of 3rd year .................and so on.
 |
| Simple interest formula |
Amount = Principal + Interest
What is compound interest
Interest earned on both the principal and accumulated interest of prior periods.
Difference between simple interest and compound interest:
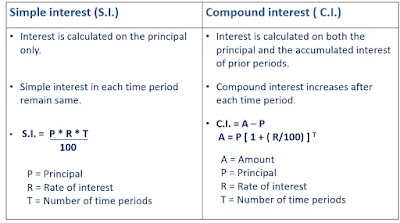 |
| difference between SI and CI |
A person takes a loan of Rs 100000 from the bank at an interest rate of 10 % per year. Find the simple interest and compound interest for the 1st and 2nd year separately.
Here, P = 100000
R = 10 % per year
For the 1st year:
| Simple interest = | P * R * T |
| 100 |
| = | 100000 * 10 * 1 |
| 100 |
= 10000
Compound Interest:
CI = A - P
So, First, we need to find the Amount
CI = A - P
So, First, we need to find the Amount
A= P * [ 1 + ( R / 100) ]T
= 100000 * [ 1 + ( 10 / 100) ]1
= 100000 * [ 1 + 0.1 ] = 110000
CI = 110000 - 100000 = 10000
Readers should note that for the first year both simple interest and compound interest are the same.
For the 2nd year:
Simple interest doesn't count interest on interest so, the principal remains the same as of 1st year.
P = 100000R = 10 %
T = 1 year
| Simple interest = | P * R * T |
| 100 |
| = | 100000 * 10 * 1 |
| 100 |
Compound interest of 2nd year
It is the simple interest earned in 2nd year on the principal and interest of 1st year. So, Amount after 1st year will become the principal for the 2nd year.
P = 110000
R = 10 %
T = 1 year
Simple interest = P * R * T 100
= 110000 * 10 * 1 100
= 11000
We can cross-check our answer using the compound interest formula:
CI = A - P
A = P * [ 1 + ( R / 100)]T
| Simple interest = | P * R * T |
| 100 |
| = | 110000 * 10 * 1 |
| 100 |
We can cross-check our answer using the compound interest formula:
CI = A - P
A = P * [ 1 + ( R / 100)]T
= 110000 * [ 1 + ( 10 / 100)]1
= 110000 * [ 1 + 0.1 ]
= 110000 * 1.1
= 121000
CI = A - P
CI = 121000 - 110000 =
CI = 11000
= 121000
CI = A - P
CI = 121000 - 110000 =
CI = 11000
The readers must note that the compound interest of 1st year was Rs 10000 and compound interest of 2nd year is 11000 rupees. the extra 1000 rupees in 2nd year are coming from the interest on the 10000 rupees ( Interest of 1st year ).
Compound interest of 2nd year:
10% of 100000 + 10 % of interest on 10000
= 10000 + 1000
= 11000
Now we know the simple interest and compound interest formula, let us solve some questions.
Jyoti invested an amount of 10000 in a bank for 2 years at an interest rate of 5 % anually compounded annually. How much Jyoti will get at the end of two years.
Answer:
CI = A - P
Rate = 5 % annually compounded annually
T ( no. of time periods) = 2/1 = 2
A = 10000 * [ 1 + (5 / 100)]2
A = 10000 * [ 1.05]2
Compound interest of 2nd year:
10% of 100000 + 10 % of interest on 10000
= 10000 + 1000
= 11000
Now we know the simple interest and compound interest formula, let us solve some questions.
Question no. 1:
Jyoti invested an amount of 10000 in a bank for 2 years at an interest rate of 5 % anually compounded annually. How much Jyoti will get at the end of two years.
Answer:
CI = A - P
A = P * [ 1 + ( R / 100)]T
= P = 10000
Rate = 5 % annually compounded annually = P = 10000
T ( no. of time periods) = 2/1 = 2
A = 10000 * [ 1 + (5 / 100)]2
A = 10000 * [ 1.05]2
A = 11025
CI = 11025 - 10000 = 1025
Note: This interest is combined interest of both 1st and 2nd year.
Questions no. 2:
Jyoti invested an amount of 10000 in a bank for 2 years at an interest rate of 5 % annually compounded monthly. How much Jyoti will get at the end of two years.
Answer:
In the above question interest rate was compounded annually but in this question interest rate is compounded monthly.
P = 10000
Rate = 5 % annually compounded monthly
= 5/12 % monthly compounded monthly
T ( Total no. of time periods) = Total no. of months in 2 year = 24
A = 10000 * [ 1 + {5 / (12 * 100)}]24
A = 10000 * [ 1.00416]24
Rate = 5 % annually compounded monthly
= 5/12 % monthly compounded monthly
T ( Total no. of time periods) = Total no. of months in 2 year = 24
A = 10000 * [ 1 + {5 / (12 * 100)}]24
A = 10000 * [ 1.00416]24
A = 11049
CI = 11049 - 10000 = 1049
CI = 11049 - 10000 = 1049
Question no. 3:
The difference in simple interest and compound interest on a certain sum of money in 2 years at 10% per year is Rs 100. find the sum.
Answer:
CI = A - P
CI = P * [ 1 + ( R / 100) ]T- P
SI = P * R * T / 100
Let 'D' be the difference of CI and SI
CI - SI = D
D = P * [ 1 + ( R / 100) ]T- P- (P * R * T )/100
D = P { [ 1 + ( R / 100) ]T- 1- (R * T) / 100 }
Given, D = 100
R = 10 %
T = 2 years
100 = P { [ 1 + ( 10 / 100) ]2- 1- (10 * 2) / 100 }
100 = P { [ 1.1 ]2- 1- 0.2 }
100 = P * 0.01
P = 100 / 0.01
P = 10000
Question no. 4:
A sum of Rs 25000 amounts to 31000 in 4 years at some rate of simple interest. find the rate percent.
Solution:
Solution:
| Simple interest ( SI ) = | P * R * T |
| 100 |
T = 4 Years
Amount = 31000
SI = 31000 - 25000 = 6000
| 6000 = | 25000 * R * 4 |
| 100 |
| R = | 100 * 6000 |
| 4 * 25000 |
R = 6 % |
Tags: Simple interest problems, compound interest problems, SI and CI
Please like, share and follow this blog. Don't forget to give feedback in the comment section. Your feedback is most important for us.
If you have any queries ask in the comment section.
difference between Simple and compound interest- formula and problems
 Reviewed by goodinfo
on
October 26, 2019
Rating:
Reviewed by goodinfo
on
October 26, 2019
Rating:
 Reviewed by goodinfo
on
October 26, 2019
Rating:
Reviewed by goodinfo
on
October 26, 2019
Rating:







No comments: